Selected Publications

Michael Ware (et al.)
Correlated photons can be used to directly measure the detection efficiency of photon counting detectors without any ties to externally calibrated standards. An overview of the history of this technique is given and the paper reviews how to implement it in a practical lab setting. Some of the sources of uncertainty in the technique and how they can be minimized and quantified are discussed. The intent is to provide the information necessary to encourage the movement of this technique from the metrology lab into the general photon-counting detector community.
Michael Ware (et al.)
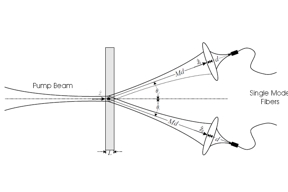
Many quantum computation and communication schemes require, or would significantly benefit from, true sources of single photon on-demand (SPOD). Unfortunately, such sources do not exist. It is becoming increasingly clear that coupling photons out of a SPOD source will be a limiting factor in many SPOD implementations. In particular, coupling these source outputs into optical fibers (usually single mode fibers) is often the preferred method for handling this light. We investigate the practical limits to this coupling as relates to parametric downconversion, an important starting point for many SPOD schemes. We also explored whether it is possible to optimize the engineering of the downconversion sources to improve on this coupling. We present our latest results in this area.

Michael J. Ware (et al.)
We present the status of our work implementing a single photon on-demand source based on a multiplexed arrangement of parametric downconverters. An array of downconverters with multiplexed outputs makes it possible to create light pulses with increased probability of containing a single photon, while suppressing the probability of producing more than one photon. This is crucial for quantum cryptographic applications. Our current setup implements the scheme in a greatly simplified manner that produces photons along with a measure of the likelihood that the light pulse emitted is just a single photon. This implementation uses a virtual array of downconverters and an array of staggered length optical fibers allowing a single detector to measure a herald photon output by a series of downconverters. This single detector arrangement is a great savings considering the cost of such detectors. The timing of the herald tells us which path the herald took, which in turn, provides information on the single vs. multiphoton probabilities. So far, our work shows that the individual correlated photon peaks are clearly resolvable with our 2.4 ns delay line steps and the 1 ns full width half maximum (FWHM) of the correlated photon peaks, and that we can observe four correlated photon peaks simultaneously, a requirement to fully implement our scheme. Our current efforts are to increase the brightness and utility of the system for incorporation into a quantum communication testbed.